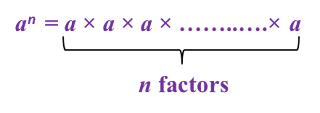1.1 Indices - Mathematics

4.1 Indices

1.1.1 Indices

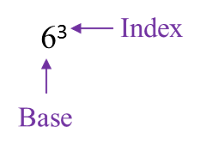
1. A number expressed in the form aⁿ is known as an index notation.

2. aⁿ is read as ‘a to the power of n’ where a is the base and n is the index.

Example:

3. If a is a real number and n is a positive integer, then

4. The value of a real number in index notation can be found by repeated multiplication.

Example:

6⁴= 6 × 6 × 6 × 6

= 1296

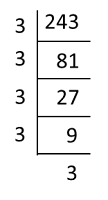
5. A number can be expressed in index notation by dividing the number repeatedly by the base.

Example:

243 = 3 × 3 × 3 × 3 × 3

= 3⁵

1.1.2 Multiplication of Numbers in Index Notation

The multiplication of numbers or algebraic terms with the same base can be done by using the Law of Indices.

a^m× aⁿ= a^m^{+ n}

Example:

3³× 3⁸= 3³⁺⁸

= 3¹¹

1.1.3 Division of Numbers in Index Notation

1. The Law of indices for division is:

a^m÷ aⁿ= a^m^{- n}

Example:

4¹²÷ 4¹²= 4^12-12

= 4⁰

= 1

2. a⁰= 1

1.1.4 Raise Numbers and Algebraic Terms in Index Notation to a Power

To raise a number in index notation to a power, multiply the two indices while keeping the base unchanged.

(a^m)ⁿ= (aⁿ)^m= a^mⁿ

Example:

(4³)⁷ = 4³×⁷

= 4²¹

1.1.5 Negative Indices

a^{- n} = \frac{1}{a^{n}}

Example:

5^{- 2} = \frac{1}{5^{2}} = \frac{1}{25}

1.1.6 Fractional Indices

a^{\frac{m}{n}} = \sqrt[n]{a^{m}} or {(\sqrt[n]{a})}^{m}

Example:

\begin{array}{l} 64^{\frac{2}{3}} = \sqrt[3]{64^{2}} or {(\sqrt[3]{64})}^{2} \\ = 16 \end{array}