Question 10:
Diagram below shows two quadrants, AOC and EOD with centre O.
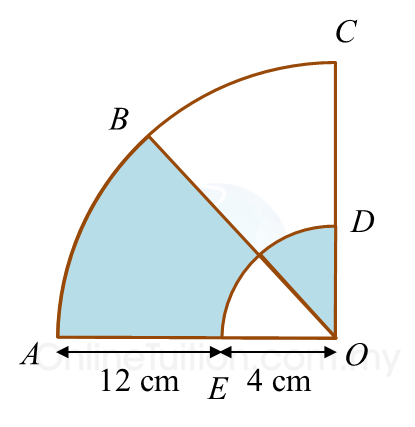
Sector AOB and sector BOC have the same area.
Calculate the area, in cm2, of the coloured region.
(Use π=227)
Solution:
Area of the sector AOB=Area of the sector BOCTherefore, ∠AOB=∠BOC=90o÷2=45oArea of the coloured region=45o360o×227×162=10047 cm2
Diagram below shows two quadrants, AOC and EOD with centre O.

Sector AOB and sector BOC have the same area.
Calculate the area, in cm2, of the coloured region.
(Use π=227)
Solution:
Area of the sector AOB=Area of the sector BOCTherefore, ∠AOB=∠BOC=90o÷2=45oArea of the coloured region=45o360o×227×162=10047 cm2
Question 11:
The Town Council plans to build an equilateral triangle platform in the middle of a roundabout. The diameter of circle RST is 24 m and the perpendicular distance from R to the line ST is 18 m. as shown in Diagram below.
 Find the perimeter of the platform.
Find the perimeter of the platform.
Solution:
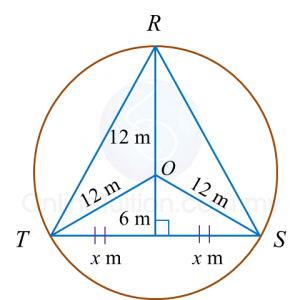
Given diameter = 24 m
hence radius = 12 m
O is the centre of the circle.
Using Pythagoras’ theorem:
x2=122−62x=√144−36 =10.39 mTS=RS=RT =10.39 m ×2 =20.78 mPerimeter of the platformTS+RS+RT=20.78×3=63.34 m
The Town Council plans to build an equilateral triangle platform in the middle of a roundabout. The diameter of circle RST is 24 m and the perpendicular distance from R to the line ST is 18 m. as shown in Diagram below.
 Find the perimeter of the platform.
Find the perimeter of the platform.Solution:

Given diameter = 24 m
hence radius = 12 m
O is the centre of the circle.
Using Pythagoras’ theorem:
x2=122−62x=√144−36 =10.39 mTS=RS=RT =10.39 m ×2 =20.78 mPerimeter of the platformTS+RS+RT=20.78×3=63.34 m