Question 7:
In diagram below, AEC is a right-angled triangle with an area of 54 cm2 and BCDF is a rectangle.
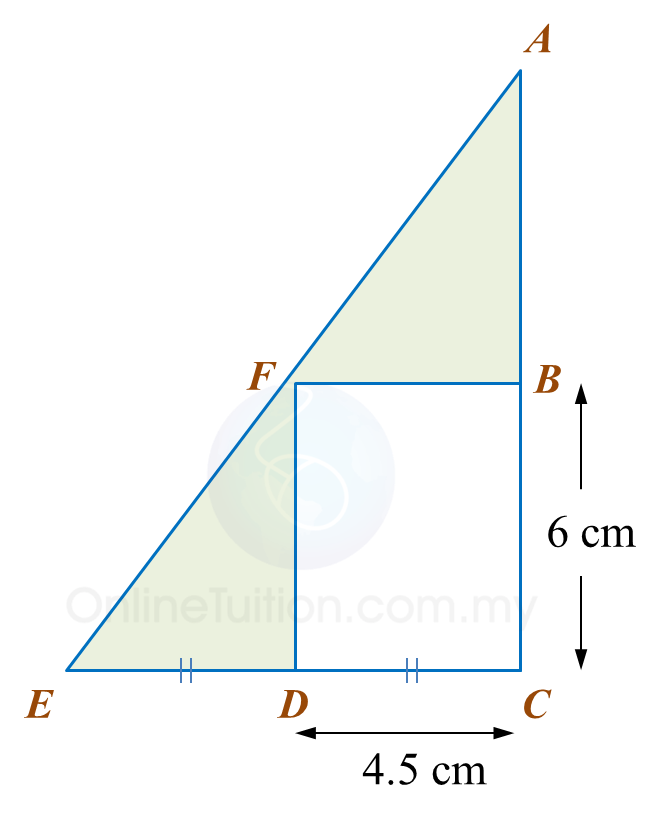 Calculate
Calculate
(a) the perimeter, in cm, of the coloured region.
(b) the area, in cm2, of the coloured region.
Solution:
(a)Given area of △ ACE12×AC×9=54 AC=54×29 AC=12 cmUsing Pythagoras’ theorem:AE=√92+122 =15 cmPerimeter of coloured region=6+4.5+6+4.5+15=36 cm
(b)Area of the coloured region=Area of △ ACE−Area of rectangle BCDF=54−(6×4.5)=54−27=27 cm2
In diagram below, AEC is a right-angled triangle with an area of 54 cm2 and BCDF is a rectangle.
 Calculate
Calculate(a) the perimeter, in cm, of the coloured region.
(b) the area, in cm2, of the coloured region.
Solution:
(a)Given area of △ ACE12×AC×9=54 AC=54×29 AC=12 cmUsing Pythagoras’ theorem:AE=√92+122 =15 cmPerimeter of coloured region=6+4.5+6+4.5+15=36 cm
(b)Area of the coloured region=Area of △ ACE−Area of rectangle BCDF=54−(6×4.5)=54−27=27 cm2
Question 8:
Diagram below shows a trapezium ABCDE. ABGF is a square with an area of 36 cm2.
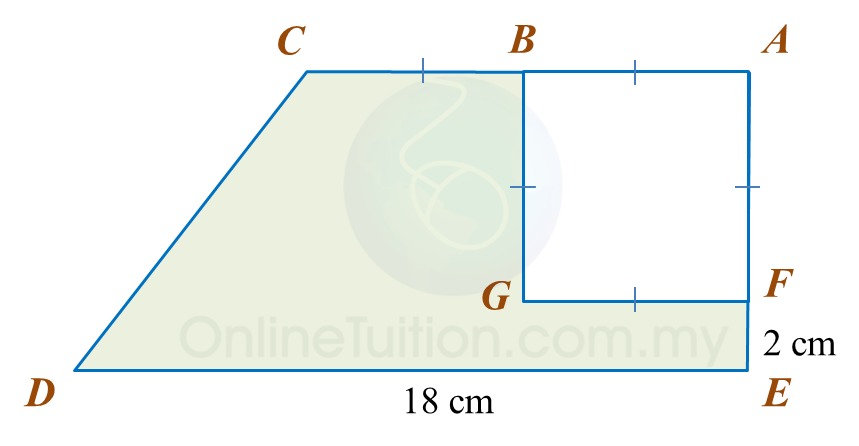
Calculate
(a) the perimeter, in cm, of the coloured region.
(b) the area, in cm2, of the coloured region.
Solution:
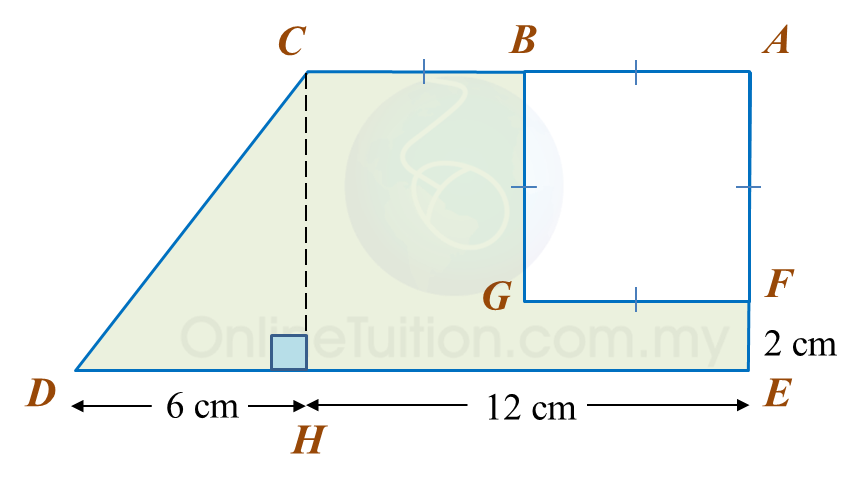
(a)Using Pythagoras’ theorem:In △ CDH,CD=√82+62 =10 cmAB=BG=GF=FA=√36=6 cmPerimeter of coloured region=6+10+18+2+6+6=48 cm
(b)Area of the coloured region=Area of trapezium ABCDE−Area of square ABGF=[12(12+18)×8]−36=[12×30×8]−36=120−36=84 cm2
Diagram below shows a trapezium ABCDE. ABGF is a square with an area of 36 cm2.

Calculate
(a) the perimeter, in cm, of the coloured region.
(b) the area, in cm2, of the coloured region.
Solution:

(a)Using Pythagoras’ theorem:In △ CDH,CD=√82+62 =10 cmAB=BG=GF=FA=√36=6 cmPerimeter of coloured region=6+10+18+2+6+6=48 cm
(b)Area of the coloured region=Area of trapezium ABCDE−Area of square ABGF=[12(12+18)×8]−36=[12×30×8]−36=120−36=84 cm2
Terima kasih banyak banyak