Question 4:
In diagram below, PQUV is a square, QRTU is a rectangle and RST is an equilateral triangle.
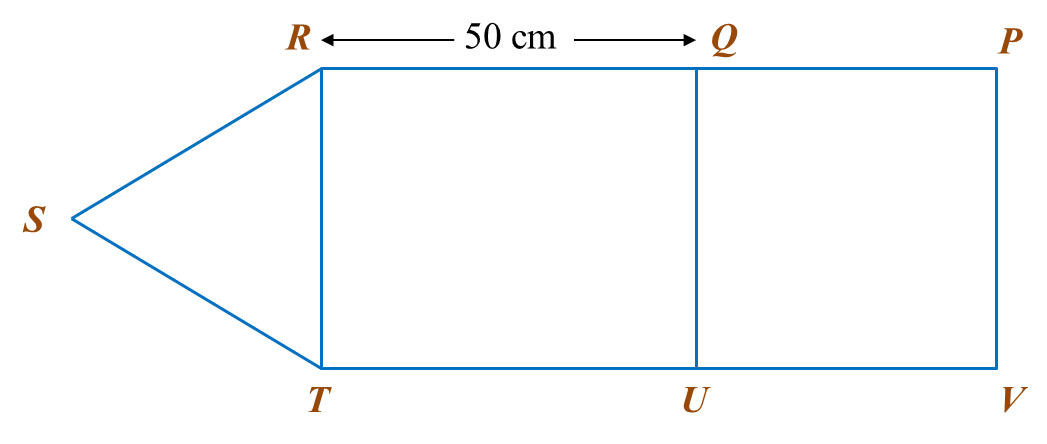
The perimeter of the whole diagram is 310 cm.
Calculate the length, in cm, of PV.
Solution:
PV=VU=TS=SR=QPGiven perimeter of the whole diagram=310 cmPV+VU+UT+TS+SR+RQ+QP=310PV+PV+50+PV+PV+50+PV=3105PV+100=310 5PV=210 PV=42 cm
In diagram below, PQUV is a square, QRTU is a rectangle and RST is an equilateral triangle.

The perimeter of the whole diagram is 310 cm.
Calculate the length, in cm, of PV.
Solution:
PV=VU=TS=SR=QPGiven perimeter of the whole diagram=310 cmPV+VU+UT+TS+SR+RQ+QP=310PV+PV+50+PV+PV+50+PV=3105PV+100=310 5PV=210 PV=42 cm
Question 5:
In diagram below, ABCD and CGFE are rectangles. M, G, E and N are midpoints of AB, BC, CD and DA respectively.
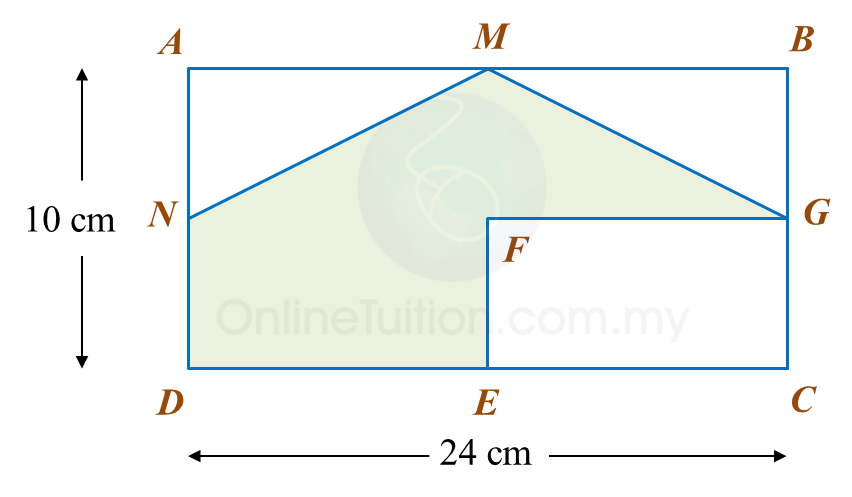
Calculate the perimeter, in cm, of the coloured region.
Solution:
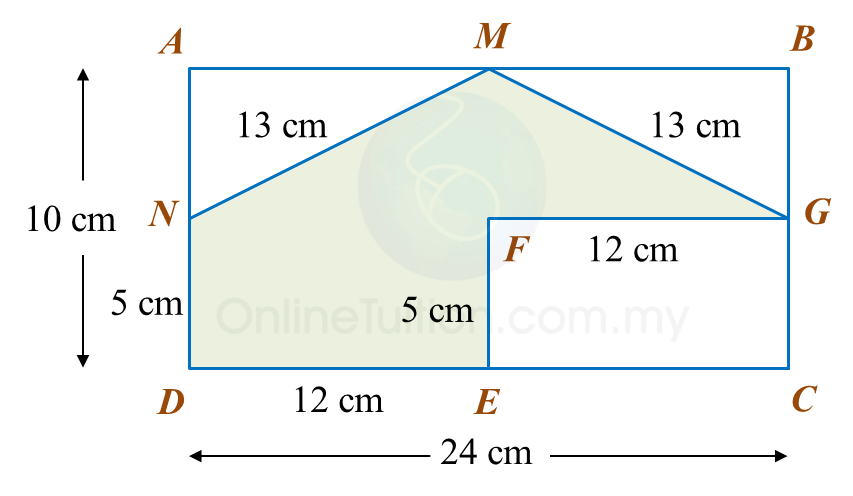
Using Pythagoras‘ theoremMG2=MF2+FG2=52+122=25+144=169MG=13 cmPerimeter of the coloured region=13+13+5+12+5+12=60 cm
In diagram below, ABCD and CGFE are rectangles. M, G, E and N are midpoints of AB, BC, CD and DA respectively.

Calculate the perimeter, in cm, of the coloured region.
Solution:

Using Pythagoras‘ theoremMG2=MF2+FG2=52+122=25+144=169MG=13 cmPerimeter of the coloured region=13+13+5+12+5+12=60 cm
Question 6:
Diagram below shows a trapezium BCDE and a parallelogram ABEF. ABC and FED are straight lines.
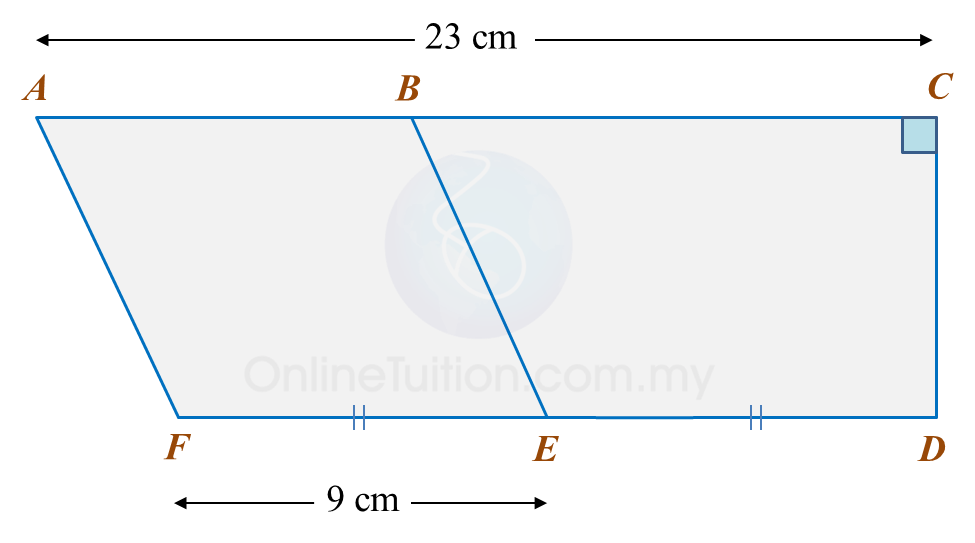 The area of ABEF is 72 cm2.
The area of ABEF is 72 cm2.
Calculate the area, in cm2, of trapezium BCDE.
Solution:
Base × Height =Area of ABFE9 cm × Height=72 cm2 Height=729=8 cmCD=8 cmBC=23−9 =14 cmArea of trapezium BCDE=12×(BC+ED)×CD=12×(14+9)×8=92 cm2
Diagram below shows a trapezium BCDE and a parallelogram ABEF. ABC and FED are straight lines.
 The area of ABEF is 72 cm2.
The area of ABEF is 72 cm2.Calculate the area, in cm2, of trapezium BCDE.
Solution:
Base × Height =Area of ABFE9 cm × Height=72 cm2 Height=729=8 cmCD=8 cmBC=23−9 =14 cmArea of trapezium BCDE=12×(BC+ED)×CD=12×(14+9)×8=92 cm2